Welcome to our group homepage!
Our group studies collective phenomena in quantum many-body systems that arise from the
intricate interplay of topology, strong correlations, and spin-orbit coupling.
In our work we typically complement analytical
approaches by a variety of sophisticated numerical techniques that exploit concepts
from statistical physics, quantum information theory, and computer science.
We are embedded in a lively research environment at the
Institute for Theoretical Physics
in Cologne and part of a number of collaborative research and funding
initiatives together with our experimental colleagues in the
Physics Department.
Research highlights
- Emergent Majorana liquids in the honeycomb Floquet code & random measurement-only Kitaev models
Floquet code: arXiv:2311.08450, random circuit: arXiv:2409.02171, Phys. Rev. Research (2024)
- Nishimori's cat — stable long-range entanglement in monitored quantum circuits
experiment: Nature Physics (2024), theory: Phys. Rev. Lett. 131, 200201 (2023)
- Transmon platform for quantum computing challenged by chaotic fluctuations
Nature Comm. 13, 2495 (2022)
- Scalable Neural Decoder for Topological Surface Codes
Phys. Rev. Lett. 128, 080505 (2022)
- Half-integer quantized anomalous thermal Hall effect in α-RuCl3
Science 373, 568 (2021)
Fun stuff
-
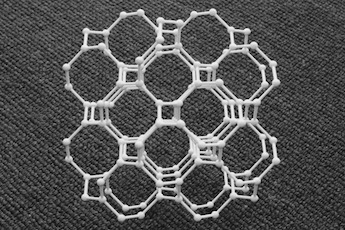
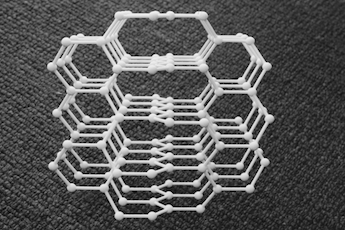
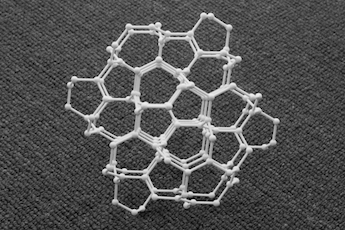
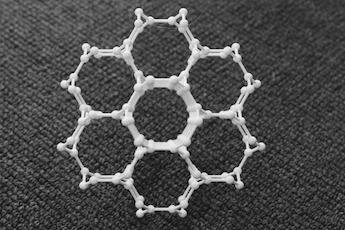
These three-dimensional tricoordinated lattice structures are underlying our recent studies of 3D Kitaev models,
which we have shown to host an unusually rich variety of Z2 spin liquids that are all analytically tractable. Examples
include gapless spin liquids with Majorana Fermi surfaces or topologically protected Weyl nodes,
as discussed in this complete classification.
You can get three-dimensional prints of these models (depicted above) from shapeways (more cool models here) — unfortunately, shapeways recently altered their pricing structure resulting in an almost 10-fold increase of printing costs for these objects.
If you want to further delve into these lattices, check out these VESTA files: (10,3)a, (10,3)b, (10,3)c, (9,3)a, (8,3)a, (8,3)b, (8,3)c, and (8,3)n.
Upcoming conference
